 Figure 1: Illustration of the lab frame and center-of-mass frame, drawn to satisfy classical addition of the velocities if the masses are set to 1. Relativistic velocity addition is not so simple.
Figure 1: Illustration of the lab frame and center-of-mass frame, drawn to satisfy classical addition of the velocities if the masses are set to 1. Relativistic velocity addition is not so simple.
Consider a particle, with mass m2 and kinetic energy Tk, incident upon a particle with mass m1 at rest. The reaction of this two-particle system to create a new two-particle system with masses m3 and m4 is expressed with the notation
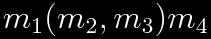
It is common to call m2 and m3 the projectile and ejectile; similarly, m1 and m4 are known as the target and recoil. The four-momentum squared is invariant:

So the Lorentz invariant quantity for the initial two-particle system is
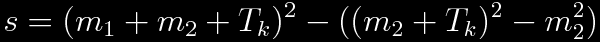 .
.
Algebraic manipulation gives the simplified result,
 .
.
Big accelerators, in pairs
Note that if identical particles were to collide with equal and opposite momenta in the lab frame, the portion of the invariant mass available for reactions (the second term in the previous equation) would go like 4 (mTk +Tk2) instead of 2mTk, which is why the biggest particle accelerators like Fermilab and CERN collide opposing beams of particles instead of a single beam on a stationary target. In other words, at energies small compared to the mass of the particles, you get twice the invariant mass for twice the accelerator. But as the energies become comparable to or greater than the mass, the factor of 4Tk2 results in big gains. For protons accelerated to 1 GeV, this amounts to a total factor of four for two accelerators compared to one. But at an energy of 1 TeV for protons, this amounts to a factor of two thousand! That's right, it's not 4 for the price of 2, it's 2000 for the price of 2! It's like we can't afford not to build bigger accelerators! (In pairs, of course.)
However, I do nuclear physics experiments, where we play different games than the particle physicists. One is to make precise measurments at low energies that are relevant to astrophysics: for reactions in stars, novae, supernovae, and big bang cosmology. Another is to perform experiments with beams of radioactive nuclei, to study exotic nuclei to further our understanding of nuclear structure. So enough of this particle physics talk about big accelerators!
In the center-of-mass frame, the momenta of m1 and m2 are equal and opposite. The invariant s does not change:

Since s is just a constant, this equation determines pcm.

pcm is the momentum of both particles in the center-of-mass frame. To determine the rapidity of the center-of-mass frame, we consider that a boost to the target with the cm rapidity gives the taget the momentum pcm.

A simple fact about the hyperbolic trig functions,
 ,
,
allows us to calculate the cm rapidity in a nice closed form:
 .
.
Given the invariant mass and the rapidity in the cm frame, it is simple to calculate the energies of the reaction products as a function of angle. The cm momentum of the reaction products follows from the previous equations, but with m1 and m2 replaced by m3 and m4:

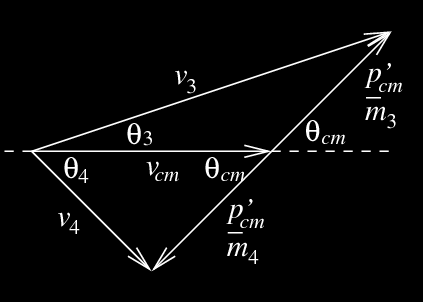 Figure 2: Triangles formed by the reaction momenta and angles in the cm and lab frames. The picture is strictly classical, using Galilean addition of the velocities, and
v3 and
v4 in the cm frame (on the right side of the triangle)
would be equal and opposite if and only if
m3 equals
m4.
Figure 2: Triangles formed by the reaction momenta and angles in the cm and lab frames. The picture is strictly classical, using Galilean addition of the velocities, and
v3 and
v4 in the cm frame (on the right side of the triangle)
would be equal and opposite if and only if
m3 equals
m4.
Given Ek and all the masses, p'cm is just a number. Applying a boost with the cm rapidity gives the momenta and energies of the products as a function of the cm angle.
The only undetermined parameter is the cm angle. The game we usually play when doing nuclear physics experiments is to determine the probability for a given reaction to happen, either as a function of the cm energy, or as a function of the cm angle, or both.
All measurements are made with detectors in a laboratory environment, so it is important to convert readily between cm and lab quantities.
Inspecting the simplified figure on the right, all the signs for the boost to the ejectile are positive. The ejectile energy and momenta in the lab are
 ,
,
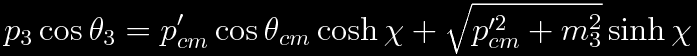 , and
, and
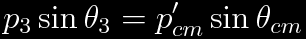 .
.
There is a minus sign to consider for the boost to the recoil. The recoil energy and momenta in the lab are
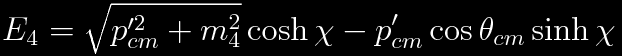 ,
,
 , and
, and
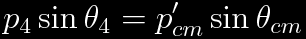 .
.
That's really all there is to it. Now, if you were deranged and masochistic, you might want to calculate one of the lab energies as a function of the lab angle. This is possible, but quite ugly. To do it, begin by rearranging the momenta so that the cm angle terms are isolated and square them both, so that addition of the squared sine and cosine terms eliminates the cm angle altogether. The remaining equations are quadratic. The form of the quadratic equation is identical for p3 and p4:

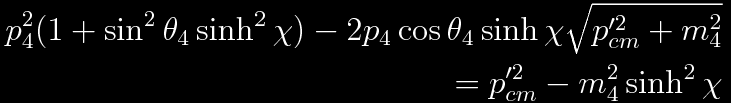
The quadratic equation gives each momentum as a function of its angle:
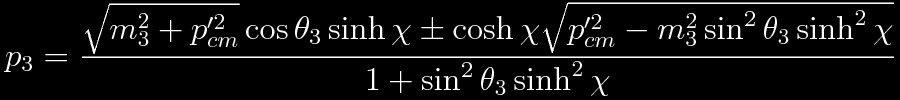

These solutions merit some discussion. The quantity under the radical in the second term of the numerator must be greater than or equal to zero, since the momentum must be real. Depending on the kinematics, this could imply a limit on the lab angle. The condition is,
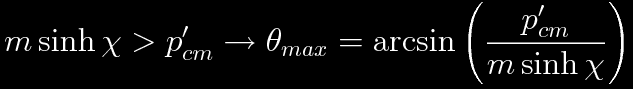
When this condition applies, the positive sign gives the high-energy reaction products, the positive and negative sign solutions become equal at the maximum lab angle (the term under the radical becomes zero), and the negative sign solution gives the low-energy reaction products. This condition usually applies for one of the reaction products in nuclear physics experiments, because beam energies are generally small compared to nuclear masses on the order of many GeV. The lighter reaction products, on the other hand, often have no maximum lab angle.
If the condition does not apply, so that there is no maximum lab angle for the given product, then the positive sign is correct. To see this, imagine that one of the outgoing particles is a photon. Taking the limit as m/p'cm goes to zero, the numerator becomes
 .
.
Since the magnitude of the momentum can never be negative, in this case the positive sign is correct.
For planning and analyzing experiments, it is often useful to convert between center-of-mass and laboratory cross sections. To do this, one must know how the solid angles vary with respect to each other. Using the relativistic transformations to write the laboratory angle as a function of the center-of-mass angle, one possible expression is
 .
.
To confuse persistent readers, the notation has been changed. pcm no longer has the apostrophe but it still refers to the center-of-mass momentum of the products. The θ without the subscript is a lab angle. And Ecm is the center-of-mass energy of the paricle in consideration.
Taking the implicit derivative of both sides of this equation, the derivative of the laboratory angle with respect to the center-of-mass angle is
 .
.
Multiply this equation by the ratio of the sines to find

This equation is for the ejectile. The only difference between the ejectile and the recoil is the minus sign, so the result is the same for the recoil with a minus sign in front of the cos(θcm) term.
To make plots of lab energy vs lab angle using these equations, try the Relativistic Kinematics Calculator for Two-Body Nuclear Reactions. Please send any questions, comments, or suggestions to Sky.
