Laplacian Operator in Parabolic Coordinates

The Laplacian Operator
from Spherical Coordinates to Parabolic Coordinates

Parabolic coordinates simplify the wave equation of an azimuthally symmetric Coulomb potential. To get from Spherical to Parabolic Coordinates, make the following substitution.

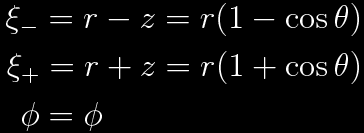
The inverse of this substitution is:

Use the chain rule to convert derivatives from the spherical coordinates to parabolic coordinates. First convert the r derivatives.

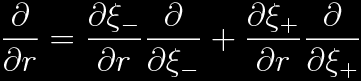
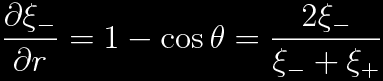
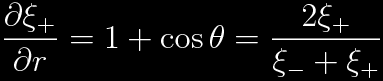
Grunt work yields a cleaner form.

Now convert the θ derivatives.

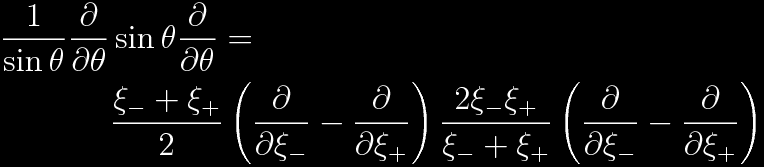
Putting the two results together and doing a little more digging yields

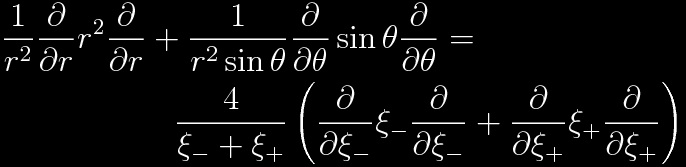
The Laplacian Operator in parabolic coordinates is:

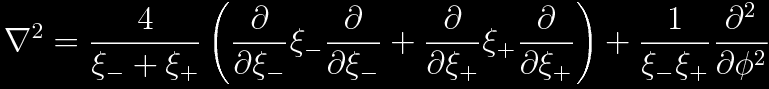
The form of the equations becomes very simple if one considers azimuthally independent solutions.

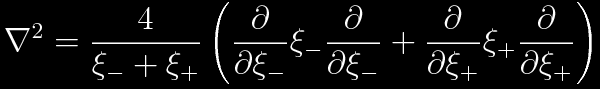
Now consider the Schrödinger Equation with a Coulomb potential in these coordinates.

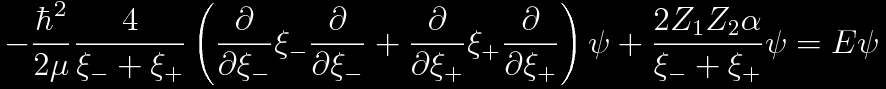
Multiplying the whole equation by the sum of the parabolic coordinates, one finds.

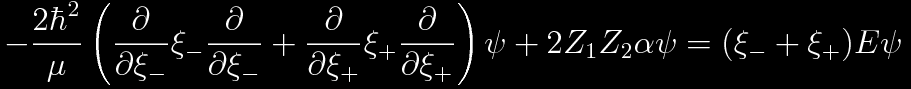
Slightly further simplified:

For many situations in physics, the desired solution must be asymptotic to a plane wave. To search for such a solution, make the following substitution.

Solving for k to eliminate the last term from the Schrödinger Equation,

,

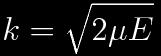the remaining function satisfies a much simpler equation:

Now make a substitution to write this equation in terms of a dimensionless coordinate.

The result is in the form of the confluent hypergeometric equation.

In the second given form of η, β is the relative velocity in units of c. I prefer to write this equation with the terms proportional to the same power of ρ grouped.